Scroll:Measurement >> Area & Perimeter of Composite Figure >> ps (2890)
Written Instructions:
For each Multiple Choice Question (MCQ), four options are given. One of them is the correct answer. Make your choice (1,2,3 or 4). Write your answers in the brackets provided..
For each Short Answer Question(SAQ) and Long Answer Question(LAQ), write your answers in the blanks provided.
Leave your answers in the simplest form or correct to two decimal places.
| 1) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 88 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
π cm2 Answer:_______________ |
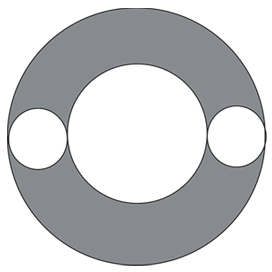
| 2) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 20 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
π cm Answer:_______________ |
| 3) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 120 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
π cm2 Answer:_______________ |
| 4) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 40 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
π cm Answer:_______________ |
| 5) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 48 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
π cm2 Answer:_______________ |
| 6) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 26 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
π cm Answer:_______________ |
| 7) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 80 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
π cm2 Answer:_______________ |
| 8) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 34 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
π cm Answer:_______________ |
| 9) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 32 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
π cm2 Answer:_______________ |
| 10) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 44 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
π cm Answer:_______________ |
| 1) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 88 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
Answer: 968 π cm2 SOLUTION 1 : Step 1: Radius of big circle → 88 cm ÷ 2 = 44 cm Step 2: Area of big circle → π r2 = π x 442 = 1936 π cm2 Step 3: Radius of medium circle → 44 cm ÷ 2 = 22 cm Step 4: Area of medium circle → π r2 = π x 222 = 484 π cm2 Step 5: Radius of small circle → 22 cm ÷ 2 = 11 cm Step 6: Area of small circle → π r2 = π x 112 = 121 π cm2 Step 7: Area of 4 small circles → 4 x 121 π cm2 = 484 π cm2 Step 8: Shaded area → 1936 π cm2 - 484 π cm2 - 484 π cm2 = 968 π cm2 |
| 2) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 20 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
Answer: 140 π cm SOLUTION 1 : Step 1: Radius of small circle → 20 cm ÷ 2 = 10 cm Step 2: Radius of big circle → 20 cm + 20 cm = 30 cm Step 3: Circumference of 2 small circles → 2 x 2 x π x 10 cm = 40 π cm Step 4: Circumference of medium circle → 2 x π x 20 cm = 40 π cm Step 5: Circumference of big circle → 2 x π x 30 cm = 60 π cm Step 6: Circumference of shaded part → 40 π cm + 40 π cm + 60 π cm = 140 π cm |
| 3) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 120 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
Answer: 1800 π cm2 SOLUTION 1 : Step 1: Radius of big circle → 120 cm ÷ 2 = 60 cm Step 2: Area of big circle → π r2 = π x 602 = 3600 π cm2 Step 3: Radius of medium circle → 60 cm ÷ 2 = 30 cm Step 4: Area of medium circle → π r2 = π x 302 = 900 π cm2 Step 5: Radius of small circle → 30 cm ÷ 2 = 15 cm Step 6: Area of small circle → π r2 = π x 152 = 225 π cm2 Step 7: Area of 4 small circles → 4 x 225 π cm2 = 900 π cm2 Step 8: Shaded area → 3600 π cm2 - 900 π cm2 - 900 π cm2 = 1800 π cm2 |
| 4) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 40 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
Answer: 280 π cm SOLUTION 1 : Step 1: Radius of small circle → 40 cm ÷ 2 = 20 cm Step 2: Radius of big circle → 40 cm + 40 cm = 60 cm Step 3: Circumference of 2 small circles → 2 x 2 x π x 20 cm = 80 π cm Step 4: Circumference of medium circle → 2 x π x 40 cm = 80 π cm Step 5: Circumference of big circle → 2 x π x 60 cm = 120 π cm Step 6: Circumference of shaded part → 80 π cm + 80 π cm + 120 π cm = 280 π cm |
| 5) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 48 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
Answer: 288 π cm2 SOLUTION 1 : Step 1: Radius of big circle → 48 cm ÷ 2 = 24 cm Step 2: Area of big circle → π r2 = π x 242 = 576 π cm2 Step 3: Radius of medium circle → 24 cm ÷ 2 = 12 cm Step 4: Area of medium circle → π r2 = π x 122 = 144 π cm2 Step 5: Radius of small circle → 12 cm ÷ 2 = 6 cm Step 6: Area of small circle → π r2 = π x 62 = 36 π cm2 Step 7: Area of 4 small circles → 4 x 36 π cm2 = 144 π cm2 Step 8: Shaded area → 576 π cm2 - 144 π cm2 - 144 π cm2 = 288 π cm2 |
| 6) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 26 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
Answer: 182 π cm SOLUTION 1 : Step 1: Radius of small circle → 26 cm ÷ 2 = 13 cm Step 2: Radius of big circle → 26 cm + 26 cm = 39 cm Step 3: Circumference of 2 small circles → 2 x 2 x π x 13 cm = 52 π cm Step 4: Circumference of medium circle → 2 x π x 26 cm = 52 π cm Step 5: Circumference of big circle → 2 x π x 39 cm = 78 π cm Step 6: Circumference of shaded part → 52 π cm + 52 π cm + 78 π cm = 182 π cm |
| 7) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 80 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
Answer: 800 π cm2 SOLUTION 1 : Step 1: Radius of big circle → 80 cm ÷ 2 = 40 cm Step 2: Area of big circle → π r2 = π x 402 = 1600 π cm2 Step 3: Radius of medium circle → 40 cm ÷ 2 = 20 cm Step 4: Area of medium circle → π r2 = π x 202 = 400 π cm2 Step 5: Radius of small circle → 20 cm ÷ 2 = 10 cm Step 6: Area of small circle → π r2 = π x 102 = 100 π cm2 Step 7: Area of 4 small circles → 4 x 100 π cm2 = 400 π cm2 Step 8: Shaded area → 1600 π cm2 - 400 π cm2 - 400 π cm2 = 800 π cm2 |
| 8) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 34 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
Answer: 238 π cm SOLUTION 1 : Step 1: Radius of small circle → 34 cm ÷ 2 = 17 cm Step 2: Radius of big circle → 34 cm + 34 cm = 51 cm Step 3: Circumference of 2 small circles → 2 x 2 x π x 17 cm = 68 π cm Step 4: Circumference of medium circle → 2 x π x 34 cm = 68 π cm Step 5: Circumference of big circle → 2 x π x 51 cm = 102 π cm Step 6: Circumference of shaded part → 68 π cm + 68 π cm + 102 π cm = 238 π cm |
| 9) The figure below shows four identical small circles, one medium circle and one big circle. The diameter of the big circle is 32 cm. The radius of the medium circle is twice the radius of each small circle. Find the shaded area in terms of π .
Answer: 128 π cm2 SOLUTION 1 : Step 1: Radius of big circle → 32 cm ÷ 2 = 16 cm Step 2: Area of big circle → π r2 = π x 162 = 256 π cm2 Step 3: Radius of medium circle → 16 cm ÷ 2 = 8 cm Step 4: Area of medium circle → π r2 = π x 82 = 64 π cm2 Step 5: Radius of small circle → 8 cm ÷ 2 = 4 cm Step 6: Area of small circle → π r2 = π x 42 = 16 π cm2 Step 7: Area of 4 small circles → 4 x 16 π cm2 = 64 π cm2 Step 8: Shaded area → 256 π cm2 - 64 π cm2 - 64 π cm2 = 128 π cm2 |
| 10) The figure below is made up of one big circle, one medium circle and two identical small circles. The radius of the medium circle is 44 cm. The ratio of the radius of the small circle to the radius of the medium circle is 1 : 2. What is the perimeter of the shaded part in the figure Give your answer in terms of π .
Answer: 308 π cm SOLUTION 1 : Step 1: Radius of small circle → 44 cm ÷ 2 = 22 cm Step 2: Radius of big circle → 44 cm + 44 cm = 66 cm Step 3: Circumference of 2 small circles → 2 x 2 x π x 22 cm = 88 π cm Step 4: Circumference of medium circle → 2 x π x 44 cm = 88 π cm Step 5: Circumference of big circle → 2 x π x 66 cm = 132 π cm Step 6: Circumference of shaded part → 88 π cm + 88 π cm + 132 π cm = 308 π cm |
.png)