Scroll:Measurement >> Area of Triangle >> ps (1515)

Written Instructions:

For each Multiple Choice Question (MCQ), four options are given. One of them is the correct answer. Make your choice (1,2,3 or 4). Write your answers in the brackets provided..

For each Short Answer Question(SAQ) and Long Answer Question(LAQ), write your answers in the blanks provided.

Leave your answers in the simplest form or correct to two decimal places.


 

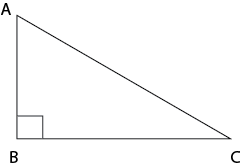
1)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 288cm.
What is the area of the triangle 




cm2


Answer:_______________




2)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 216cm.
What is the area of the triangle 




cm2


Answer:_______________




3)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 252cm.
What is the area of the triangle 




cm2


Answer:_______________




4)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 228cm.
What is the area of the triangle 




cm2


Answer:_______________




5)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 276cm.
What is the area of the triangle 




cm2


Answer:_______________




6)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 192cm.
What is the area of the triangle 




cm2


Answer:_______________




7)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 60cm.
What is the area of the triangle 




cm2


Answer:_______________




8)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 36cm.
What is the area of the triangle 




cm2


Answer:_______________




9)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 96cm.
What is the area of the triangle 




cm2


Answer:_______________




10)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 168cm.
What is the area of the triangle 




cm2


Answer:_______________




 

1)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 288cm.
What is the area of the triangle 




Answer: 3456 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 288 cm

             1 unit = 288 cm ÷ 12

                      = 24 cm

Step 2: AB → 3 units = 3 x 24 cm

                                   = 72 cm

Step 3:  BC → 4 units = 4 x 24 cm

                                   = 96 cm

Step 4: Area of triangle → 12 x 72 cm x 96 cm = 3456 cm2



2)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 216cm.
What is the area of the triangle 




Answer: 1944 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 216 cm

             1 unit = 216 cm ÷ 12

                      = 18 cm

Step 2: AB → 3 units = 3 x 18 cm

                                   = 54 cm

Step 3:  BC → 4 units = 4 x 18 cm

                                   = 72 cm

Step 4: Area of triangle → 12 x 54 cm x 72 cm = 1944 cm2



3)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 252cm.
What is the area of the triangle 




Answer: 2646 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 252 cm

             1 unit = 252 cm ÷ 12

                      = 21 cm

Step 2: AB → 3 units = 3 x 21 cm

                                   = 63 cm

Step 3:  BC → 4 units = 4 x 21 cm

                                   = 84 cm

Step 4: Area of triangle → 12 x 63 cm x 84 cm = 2646 cm2



4)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 228cm.
What is the area of the triangle 




Answer: 2166 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 228 cm

             1 unit = 228 cm ÷ 12

                      = 19 cm

Step 2: AB → 3 units = 3 x 19 cm

                                   = 57 cm

Step 3:  BC → 4 units = 4 x 19 cm

                                   = 76 cm

Step 4: Area of triangle → 12 x 57 cm x 76 cm = 2166 cm2



5)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 276cm.
What is the area of the triangle 




Answer: 3174 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 276 cm

             1 unit = 276 cm ÷ 12

                      = 23 cm

Step 2: AB → 3 units = 3 x 23 cm

                                   = 69 cm

Step 3:  BC → 4 units = 4 x 23 cm

                                   = 92 cm

Step 4: Area of triangle → 12 x 69 cm x 92 cm = 3174 cm2



6)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 192cm.
What is the area of the triangle 




Answer: 1536 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 192 cm

             1 unit = 192 cm ÷ 12

                      = 16 cm

Step 2: AB → 3 units = 3 x 16 cm

                                   = 48 cm

Step 3:  BC → 4 units = 4 x 16 cm

                                   = 64 cm

Step 4: Area of triangle → 12 x 48 cm x 64 cm = 1536 cm2



7)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 60cm.
What is the area of the triangle 




Answer: 150 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 60 cm

             1 unit = 60 cm ÷ 12

                      = 5 cm

Step 2: AB → 3 units = 3 x 5 cm

                                   = 15 cm

Step 3:  BC → 4 units = 4 x 5 cm

                                   = 20 cm

Step 4: Area of triangle → 12 x 15 cm x 20 cm = 150 cm2



8)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 36cm.
What is the area of the triangle 




Answer: 54 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 36 cm

             1 unit = 36 cm ÷ 12

                      = 3 cm

Step 2: AB → 3 units = 3 x 3 cm

                                   = 9 cm

Step 3:  BC → 4 units = 4 x 3 cm

                                   = 12 cm

Step 4: Area of triangle → 12 x 9 cm x 12 cm = 54 cm2



9)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 96cm.
What is the area of the triangle 




Answer: 384 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 96 cm

             1 unit = 96 cm ÷ 12

                      = 8 cm

Step 2: AB → 3 units = 3 x 8 cm

                                   = 24 cm

Step 3:  BC → 4 units = 4 x 8 cm

                                   = 32 cm

Step 4: Area of triangle → 12 x 24 cm x 32 cm = 384 cm2



10)  

In the right-angled triangle shown below, the ratio of the length of sides AB : BC : CA is 3 : 4 : 5.
The perimeter of the triangle is 168cm.
What is the area of the triangle 




Answer: 1176 cm2


SOLUTION 1 :

AB : BC : CA
3 : 4 : 5

 

Step 1:  3 + 4 +5 = 12 units

            12 units = 168 cm

             1 unit = 168 cm ÷ 12

                      = 14 cm

Step 2: AB → 3 units = 3 x 14 cm

                                   = 42 cm

Step 3:  BC → 4 units = 4 x 14 cm

                                   = 56 cm

Step 4: Area of triangle → 12 x 42 cm x 56 cm = 1176 cm2